12.3. Interface Velocities
The average velocity in a liquid slug is
but the presence of films on the cladding and structure causes the liquid-vapor interface to move at a somewhat different velocity. Figure 12.3.1 shows an interface moving at a velocity vi for a time interval Δt. A film of liquid sodium is present in the vapor region on both the cladding and structure. The cladding film is of thickness wfe, while that on the structure has thickness wfs. The film on the cladding can move with velocity vfe, and the structure film can have a velocity of vfs. In later versions of SASSYS-1, film motion modeling will be available, and so vfe and vfs are included in the equations below for completeness; currently, however, film motion is neglected, and vfe and vfs are set to zero.
If the coolant channel volume between zi(t) and zi(t+Δt) is taken as a control volume, then the liquid volume Vl in the control volume at time t is
where Pe is the outer perimeter of the cladding and γ2 is the ratio of the surface area of the structure to the surface area of the cladding. At t+Δt the liquid volume in the control volume is
Accounting for the liquid added to and subtracted from the control volume during Δt gives
Substituting Eq. (12.3-2) and Eq. (12.3-3) into Eq. (12.3-4) gives
or
which is the equation used for computing interface velocities. Note that this equation is applicable whether the interface is moving in the positive or the negative axial direction and holds whether the interface is moving towards the liquid side or the vapor side.
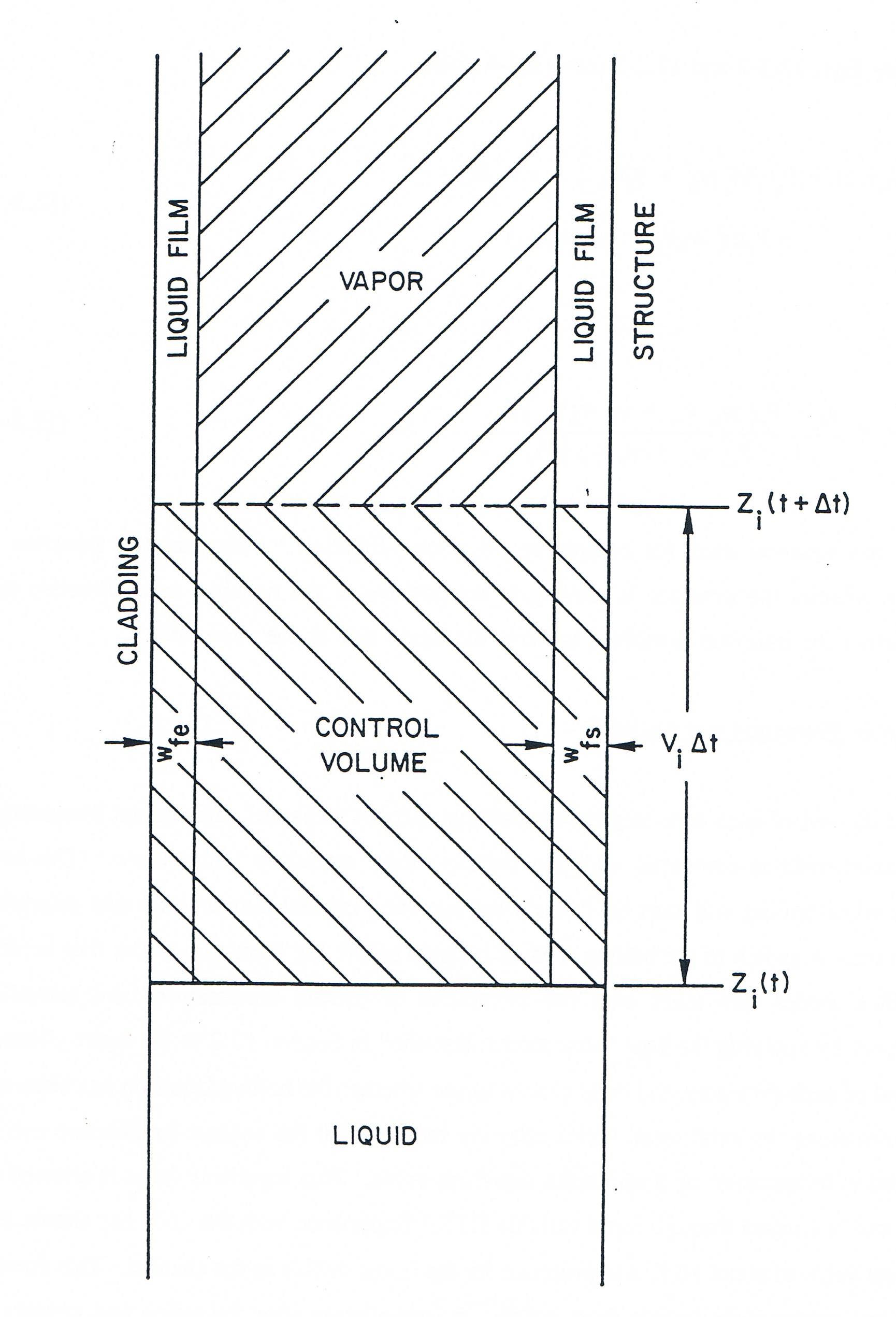
Figure 12.3.1 Geometry Associated with the Liquid-Vapor Interface Velocity Calculation